1 Derivation of Expected Covariances

To understand what it is about the observed statistics that suggests sibling interactions in our twin data we must follow through a little algebra. We shall try to keep this as simple as possible by considering the path model in Figure 8.3, which depicts the influence of an arbitrary latent

Figure 8.3: Path diagram showing influence of arbitrary exogenous variable $X$ on phenotype $P$ in a pair of relatives (for univariate twin data, incorporating sibling interaction).

variable, $X$, on the phenotype $P$. As long as our latent variables -- A, C, E, etc. -- are independent of each other, their effects can be considered one at a time and then summed, even in the presence of social interactions. The linear model corresponding to this path diagram is

$$P_1 = sP_2 + xX_1$$ (47)

$$P_2 = sP_1 + xX_2$$ (48)

Or, in matrices:

$$\left( \begin{array}{r} P_1 \\ P_2 \end{array} \right) = \le... ... \right) \left( \begin{array}{r} X_1\\ X_2 \end{array} \right)$$

which in turn we can write more economically as

$$\bf y = \bf B \bf y + \bf G \bf x$$

Following the rules for matrix algebra set out in Chapters 4 and , we can rearrange this equation, as before:

$$\bf y - \bf B \bf y = \bf G \bf x$$ (49)

$${\bf I}\bf y - \bf B \bf y = \bf G \bf x$$ (50)

$$({\bf I} - \bf B) \bf y = \bf G \bf x \; ,$$ (51)

and then, multiplying both sides of this equation by the inverse of (I - B), we have

$$\bf y = ({\bf I} -\bf B)^{-1} \bf G \bf x \; .$$ (52)

In this case, the matrix (I - B) is simply

$$\left( \begin{array}{rr} 1&-s\\ -s&1 \end{array} \right) \;,$$

which has determinant $1-s^2$, so $({\bf I} -\bf B)^{-1}$ is

$$\frac{1}{1-s^2}\otimes \left( \begin{array}{rr} 1&s\\ s&1 \end{array} \right) \; .$$

The symbol $\otimes$ is used to represent the Kronecker product, which in this case simply means that each element in the matrix is to be multiplied by the constant $\frac{1}{1-s^2}$.

We have a vector of phenotypes on the left hand side of equation 8.8. In the chapter on matrix algebra (p. ) we showed how the covariance matrix could be computed from the raw data matrix $\bf T$ by expressing the observed data as deviations from the mean to form matrix $\bf U$, and computing the matrix product $\bf UU^\prime$. The same principle is applied here to the vector of phenotypes, which has an expected mean of 0 and is thus already expressed in mean deviate form. So to find the expected variance-covariance matrix of the phenotypes $P_1$ and $P_2$, we multiply by the transpose:

$${\cal E} \left\{ \bf y\bf y'\right\} = \left\{({\bf I}-\bf B)^{-1}\bf G \bf x\right\} \left\{({\bf I}-\bf B)^{-1}\bf G \bf x\right\}^\prime$$ (53)

$$= ({\bf I}-\bf B)^{-1}\bf G {\cal E} \left\{ \bf x\bf x'\right\} \bf G'({\bf I}-\bf B)^{-1'} \; .$$ (54)

Now in the middle of this equation we have the matrix product ${\cal E} \left\{ \bf x\bf x'\right\}$. This is the covariance matrix of the x variables. For our particular example, we want two standardized variables, $X_1$ and to have unit variance and correlation $r$ so the matrix is:

$$\left( \begin{array}{rr} 1 & r\\ r& 1 \end{array} \right) \; .$$

We now have all the pieces required to compute the covariance matrix, recalling that for this case,

$$\bf G = \left( \begin{array}{rr} x & 0\\ 0& x \end{array} \right)$$ (55)

$$({\bf I}-\bf B)^{-1} = \frac{1}{1-s^2}\otimes \left( \begin{array}{rr} 1&s\\ s&1 \end{array} \right)$$ (56)

$${\cal E}\left\{ \bf x\bf x'\right\} = \left( \begin{array}{rr} 1&r\\ r&1 \end{array} \right) \; .$$ (57)

The reader may wish to show as an exercise that by substituting the right hand sides of equations 8.11 to 8.13 into equation , and carrying out the multiplication, we obtain:

$${\cal E}\left\{ \bf x\bf x'\right\} = \frac{x^2}{(1-s^2)^2}\otimes \left( \begin{array}{rr} 1+2sr+s^2& r+2s+rs^2\\ r+2s+rs^2 & 1+2sr+s^2 \end{array} \right)$$ (58)

We can use this result to derive the effects of sibling interaction on the variance and covariance due to a variety of sources of individual differences. For example, when considering:

1. additive genetic influences, $x^2=a^2$ and $r=\alpha$, where $\alpha$ is 1.0 for MZ twins and 0.5 for DZ twins;
2. shared environment influences, $x^2=c^2$ and $r=1$;
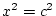3. non-shared environmental influences, $x^2=e^2$ and $r=0$;
4. genetic dominance, $x^2=d^2$ and $r=\delta$, where $\delta=1.0$ for MZ twins and $\delta=0.25$ for DZ twins.

These results are summarized in Table 8.3.

Table 8.3: The effects of sibling interaction(s) on variance and covariance components between pairs of relatives.

Source	Variance	Covariance
Additive genetic	$\omega (1+2s \alpha +s^2)a^2$	$\omega (\alpha+2s+\alpha s^2)a^2$
Dominance genetic	$\omega (1+2s \delta +s^2)d^2$	$\omega (\delta+2s+\delta s^2)d^2$
Shared environment	$\omega (1+2s+s^2)c^2$	$\omega (1+2s+s^2)c^2$
Non-shared environment	$\omega (1+s^2)e^2$	$\omega 2se^2$
$\omega$ represents the scalar $\frac{1}{(1-s^2)^2}$ obtained from equation 8.14.