Mx Script for Phenotypic Factor Analysis of Four Variables

Single factor phenotypic model: 4 arithmetic computation variables
 Data NGroups=1 NInput_vars=4 NObservations=42 
 CMatrix
  259.664 
  209.325 259.939 
  209.532 220.755 245.235 
  221.610 221.491 221.317 249.298 
 Labels Time1 Time2 Time3 Time4 
 Begin Matrices;
  B Full 1 4 Free
  P Symm 1 1
  E Diag 4 4 Free
 End Matrices;
  Value 1 P 1 1 
  Start 9 All
 Covariances B*P*B'+E; 
 Option RSiduals
End

The parameters in the group type statement indicate that we have only NGroup=1 group (consisting of NObservations=42 subjects) and there are NInput_vars=4 input variables. The loadings of the four variables on the single common factor are estimated in matrix B and their specific variances are estimated on the diagonal of matrix E. In this phenotypic factor model, we have sufficient information to estimate factor loadings and specific variances for the four variables, but we cannot simultaneously estimate the variance of the common factor because the model would then be underidentified. We therefore fix the variance of the latent factor to an arbitrary non-zero constant, which we choose to be unity in order to keep the factor loadings and specific variances in the original scale of measurement (+Value 1 P 1 1+). The Mx output (after editing) from this common factor model is shown below. The PARAMETER SPECIFICATIONS section illustrates the assignment of parameter numbers to matrices declared Free in the matrices section. Consecutive parameter numbers are given to free elements in matrices in the order in which they appear. It is always advisable to check the parameter specifications for the correct assignment of free and constrained parameters. The output depicts the single common factor structure of the model: there are free factor loadings for each of the four variables on the common factor, and specific variance parameters for each of the observed variables. Thus, the model has a total of 8 parameters to explain the 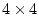$4(4+1)/2 = 10$ free statistics. The results - from the MX PARAMETER ESTIMATES section of the Mx output - are summarized in Table 10.2.3. The chi-squared goodness-of-fit value of 1.46 for 2 degrees of freedom suggests that this single factor model adequately explains the observed covariances (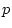$p$ = .483). This also may be seen by comparing the elements of the fitted covariance matrix and the observed covariance matrix, which are seen to be very similar. The fitted covariance matrix is printed by Mx when the RSiduals option is added. The fitted covariance matrix is calculated by Mx using expression 10.2 with the final estimated parameter values.