



Next: 3 Within Family Differences
Up: 2 Heredity and Variation
Previous: 5 Variation and Modification
Index
Look at the two sets of data shown in Figure 1.2. The
first
Figure 1.2:
Two scatterplots
of weight in: a) a large sample of DZ twin pairs, and b)
pairs of individuals matched at random.
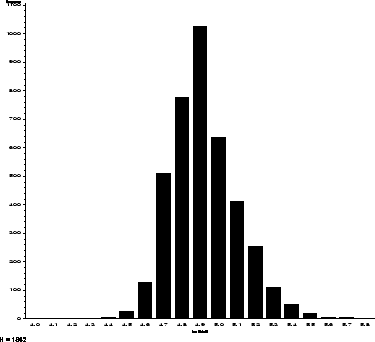 |
part of the figure is a scatterplot of measurements of weight in a
large sample of non-identical (fraternal, dizygotic, DZ)
twins.
Each cross in the diagram
represents a single twin pair. The second part of the figure is a
scatterplot of pairs of completely unrelated people from the same
population. Notice how the two parts of the figure differ. In the
unrelated pairs the pattern of crosses gives the general impression of
being circular; in general, if we pick a particular value on the X
axis (first person's weight), it makes little difference to how heavy
the second person is on average. This is what it means to say that
measures are uncorrelated -- knowing the score of the first
member of a pair makes it no easier to predict the score of the second
and vice-versa. By comparison, the scatterplot for the
fraternal twins (who are related biologically to the same degree as
brothers and sisters) looks somewhat different. The pattern of crosses is
slightly elliptical and tilted upwards. This means that as we move from
lighter first twins towards heavier first twins (increasing values on
the X axis), there is also a general tendency for the average scores
of the second twins (on the Y axis) to increase. It appears that the
weights of twins are somewhat correlated. Of course, if we take any
particular X value, the Y values are still very variable so the
correlation is not perfect. The correlation coefficient (see
Chapter 2) allows us to
quantify the degree of relationship between the two sets of measures.
In the unrelated individuals, the correlation turns out to be 0.02
which is well within the range expected simply by chance alone if
the measures were really independent. For the fraternal twins,
on the other hand, the correlation is 0.44 which is far greater
than we would expect in so large a sample if the pairs of measures
were truly independent.
The data on weight illustrate the important general point that
relatives are usually much more alike than unrelated individuals from
the same population. That is, although there are large individual
differences for almost every trait than can be measured, we find that
the trait values of family members are often quite similar.
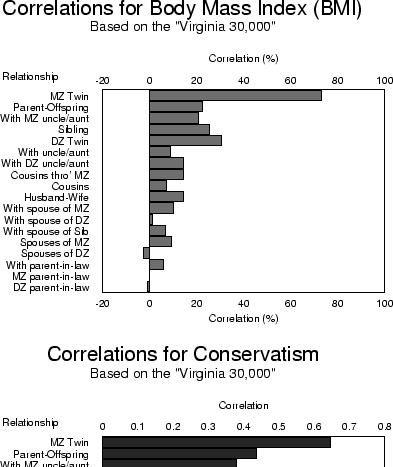
Figure 1.3:
Correlations for body mass index (weight/height ) and conservatism between
relatives. Data were obtained from large samples of nuclear families
ascertained through twins.
) and conservatism between
relatives. Data were obtained from large samples of nuclear families
ascertained through twins.
 |
Figure 1.3 gives the correlations between relatives in
large samples of nuclear families for body mass index (BMI), and
conservatism. One simple way of interpreting the correlation
coefficient is to multiply it by 100 and treat it as a percentage.
The correlation ( 100) is the ``percentage of the total
variation in a trait which is caused by factors shared by members of a
pair." Thus, for example, our correlation of 0.44 for the weights of
DZ twins implies that, of all the factors which create variation in
weight, 44% are factors which members of a DZ twin pair have in common. We
can offer a similar interpretation for the other kinds of
relationship. A problem becomes immediately apparent. Since the DZ
twins, for example, have spent most of their lives together, we cannot
know whether the 44% is due entirely to the fact that they shared the
same environment in utero, lived with the same parents after
birth, or simply have half their genes in common -- and we shall
never know until we can find another kind of relationship in which the
degree of genetic similarity, or shared environmental similarity, is
different.
Figure 1.4 gives a scattergram for the weights of a large
100) is the ``percentage of the total
variation in a trait which is caused by factors shared by members of a
pair." Thus, for example, our correlation of 0.44 for the weights of
DZ twins implies that, of all the factors which create variation in
weight, 44% are factors which members of a DZ twin pair have in common. We
can offer a similar interpretation for the other kinds of
relationship. A problem becomes immediately apparent. Since the DZ
twins, for example, have spent most of their lives together, we cannot
know whether the 44% is due entirely to the fact that they shared the
same environment in utero, lived with the same parents after
birth, or simply have half their genes in common -- and we shall
never know until we can find another kind of relationship in which the
degree of genetic similarity, or shared environmental similarity, is
different.
Figure 1.4 gives a scattergram for the weights of a large
Figure 1.4:
Scatterplot of weight in a large sample of MZ twins.
 |
sample of identical (monozygotic, MZ)
twins.
Whereas DZ twins,
like siblings, on average share only half their genes, MZ twins are
genetically identical. The scatter of points is now much more clearly
elliptical, and the 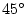 tilt of the major axis is especially
obvious. The correlation in the weights in this sample of MZ twins is
0.77, almost twice that found for DZ's. The much greater resemblance
of MZ twins, who are expected to have completely identical genes
establishes a strong prima facie case for the contribution of
genetic factors to differences in weight. One of the tasks to be
addressed in this book is how to interpret such differential patterns
of family resemblance in a more rigorous, quantitative, fashion.
tilt of the major axis is especially
obvious. The correlation in the weights in this sample of MZ twins is
0.77, almost twice that found for DZ's. The much greater resemblance
of MZ twins, who are expected to have completely identical genes
establishes a strong prima facie case for the contribution of
genetic factors to differences in weight. One of the tasks to be
addressed in this book is how to interpret such differential patterns
of family resemblance in a more rigorous, quantitative, fashion.




Next: 3 Within Family Differences
Up: 2 Heredity and Variation
Previous: 5 Variation and Modification
Index
Jeff Lessem
2002-03-21

