



Next: 2 Common Effects G
Up: 1 Models for G
Previous: 1 Models for G
Index
First we consider the general G 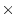 E interaction model,
similar to the general sex-limitation model discussed in
section 9.2.3. This model not only allows the magnitude of
genetic and environmental effects to vary across environmental
conditions, but also, by using information from twin pairs discordant
for environmental exposure, enables us to determine whether it is the
same set of genes or environmental features that are expressed in the
two environments. Just as we used twins who were discordant for sex
(i.e., DZO pairs) to illustrate the sex-limitation model, we use twins
discordant for environmental exposure to portray the general G
E interaction model,
similar to the general sex-limitation model discussed in
section 9.2.3. This model not only allows the magnitude of
genetic and environmental effects to vary across environmental
conditions, but also, by using information from twin pairs discordant
for environmental exposure, enables us to determine whether it is the
same set of genes or environmental features that are expressed in the
two environments. Just as we used twins who were discordant for sex
(i.e., DZO pairs) to illustrate the sex-limitation model, we use twins
discordant for environmental exposure to portray the general G
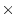 E interaction model. Before modeling genetic and
environmental effects on these individuals, one must order the twins
so that the first of the pair has not been exposed to the putative
modifying environment, while the second has (or vice versa, as
long as the order is consistent across families and across groups).
The path model for the discordant DZ pairs is then identical to that
used for the dizygotic opposite-sex pairs in the sex-limitation model;
for the discordant MZ pairs, it differs only from the DZ model in the
correlation structure of the ultimate genetic variables (see
Figure 9.3).
E interaction model. Before modeling genetic and
environmental effects on these individuals, one must order the twins
so that the first of the pair has not been exposed to the putative
modifying environment, while the second has (or vice versa, as
long as the order is consistent across families and across groups).
The path model for the discordant DZ pairs is then identical to that
used for the dizygotic opposite-sex pairs in the sex-limitation model;
for the discordant MZ pairs, it differs only from the DZ model in the
correlation structure of the ultimate genetic variables (see
Figure 9.3).
Figure 9.3:
The general genotype 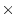 environment
interaction model for twin data. Path diagram is for MZ and DZ twins
discordant for environmental exposure. For MZ pairs,
environment
interaction model for twin data. Path diagram is for MZ and DZ twins
discordant for environmental exposure. For MZ pairs, 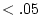 = 1.0
and
= 1.0
and 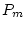 = 1.0; for DZ pairs,
= 1.0; for DZ pairs, 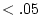 = 0.5 and
= 0.5 and 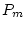 = 0.25.
The subscripts
= 0.25.
The subscripts  and
and 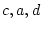 identify variables and parameters and
unexposed and exposed twins, respectively.
identify variables and parameters and
unexposed and exposed twins, respectively.
 |
Among the ultimate variables in Figure 9.3 are genetic
effects that are correlated between the unexposed and exposed twins
and those that influence only the latter (i.e., environment-specific
effects). For the concordant unexposed and concordant exposed MZ and
DZ pairs, path models are comparable to those used for female-female
and male-male MZ and DZ pairs in the sex-limitation analysis, with
environment-specific effects (instead of sex-specific effects)
operating on the exposed twins (instead of the male twins). As a
result, the model predicts equal variances within an exposure
class, across zygosity groups.
In specifying the general G 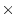 E interaction model in Mx,
one must again use boundary constraints, in order to avoid negative
covariance estimates for the pairs discordant for exposure
(Appendix
E interaction model in Mx,
one must again use boundary constraints, in order to avoid negative
covariance estimates for the pairs discordant for exposure
(Appendix ![[*]](crossref.png) ).
Unlike the general sex-limitation analysis, there is enough
information in a G
).
Unlike the general sex-limitation analysis, there is enough
information in a G 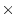 E analysis to estimate two
environment-specific effects. Thus, the magnitude of
environment-specific additive and dominant genetic or additive
genetic and common environmental effects can be determined. It still
is not possible to simultaneously estimate the magnitude of common
environmental and dominant genetic effects.
E analysis to estimate two
environment-specific effects. Thus, the magnitude of
environment-specific additive and dominant genetic or additive
genetic and common environmental effects can be determined. It still
is not possible to simultaneously estimate the magnitude of common
environmental and dominant genetic effects.




Next: 2 Common Effects G
Up: 1 Models for G
Previous: 1 Models for G
Index
Jeff Lessem
2002-03-21
![[*]](crossref.png) ).
Unlike the general sex-limitation analysis, there is enough
information in a G
).
Unlike the general sex-limitation analysis, there is enough
information in a G