



Next: 4 Multiple Genetic Factor
Up: 3 Simple Genetic Factor
Previous: 3 Fitting the Multivariate
Index
4 Fitting a Second Genetic Factor
The genetic common factor model we introduced in Sections 10.3.3
and 10.3.2 may be extended to address more specific questions about
the data. In the arithmetic computation measures, for example, it is
reasonable to hypothesize two genetic factors: one general factor
contributing to all measurements of arithmetic computation, and a second
``alcohol'' factor which influences the measures taken after the challenge
dose of alcohol. The most parsimonious extension of our common factor
model may involve the addition of only 1 free parameter which represents
each of the factor loadings on the alcohol factor (that is, the alcohol
loadings may be equated for all alcohol measurements).
The Mx script corresponds very closely to that used in
section 10.3.2, using the X for the genetic common factors We
add the latent alcohol factors for twins 1 and 2 as a second column with
the following specification statement:
Specify X
1 0
2 5
3 5
4 5
The addition of the single parameter for all alcohol loadings reflects a
model having 13 parameters and
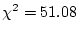 degrees of
freedom. We can, therefore, test the significance of the alcohol factor
by comparing the goodness-of-fit chi-squared value for this model with
that obtained from the model of Section 10.3.2 for a
degrees of
freedom. We can, therefore, test the significance of the alcohol factor
by comparing the goodness-of-fit chi-squared value for this model with
that obtained from the model of Section 10.3.2 for a 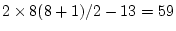 d.f.
test. Table 10.3.4 shows the results of the two-factor
multivariate genetic model.
d.f.
test. Table 10.3.4 shows the results of the two-factor
multivariate genetic model.
Table 10.6:
Parameter estimates from the two genetic factors model
| |
|
|
|
|
| |
 |
 |
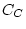 |
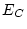 |
| Time 1 |
15.067 |
0.000 |
4.408 |
6.674 |
| Time 2 |
13.701 |
4.270 |
6.091 |
6.277 |
| Time 3 |
13.518 |
4.270 |
6.800 |
5.644 |
| Time 4 |
13.832 |
4.270 |
5.695 |
5.928 |
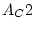 , 59 df, p=.858 , 59 df, p=.858 |
The estimated genetic factor loading for the alcohol variables (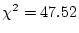 ) is
reasonably large, but much smaller than the loadings on the general
genetic factor. This difference is more apparent when we consider
proportions of genetic variance accounted for by these two factors, being
) is
reasonably large, but much smaller than the loadings on the general
genetic factor. This difference is more apparent when we consider
proportions of genetic variance accounted for by these two factors, being
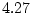 or 9% for the alcohol factor, and
or 9% for the alcohol factor, and 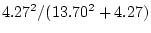 for the general genetic factor. The model yields a
for the general genetic factor. The model yields a
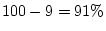 (
(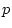 = .86), indicating a good fit to the data. The chi-squared
test for the significance of the alcohol factor loadings is
= .86), indicating a good fit to the data. The chi-squared
test for the significance of the alcohol factor loadings is
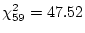 , which is not quite significant at the .05 level. Thus, while the
hypothesis of there being genetic effects on the alcohol measures
additional to those influencing arithmetic skills fits the observed data
better, the increase in fit obtained by adding the alcohol factor does not
reach statistical significance.
, which is not quite significant at the .05 level. Thus, while the
hypothesis of there being genetic effects on the alcohol measures
additional to those influencing arithmetic skills fits the observed data
better, the increase in fit obtained by adding the alcohol factor does not
reach statistical significance.




Next: 4 Multiple Genetic Factor
Up: 3 Simple Genetic Factor
Previous: 3 Fitting the Multivariate
Index
Jeff Lessem
2002-03-21